画像 level curves of a function 173846-Sketch level curves of a function

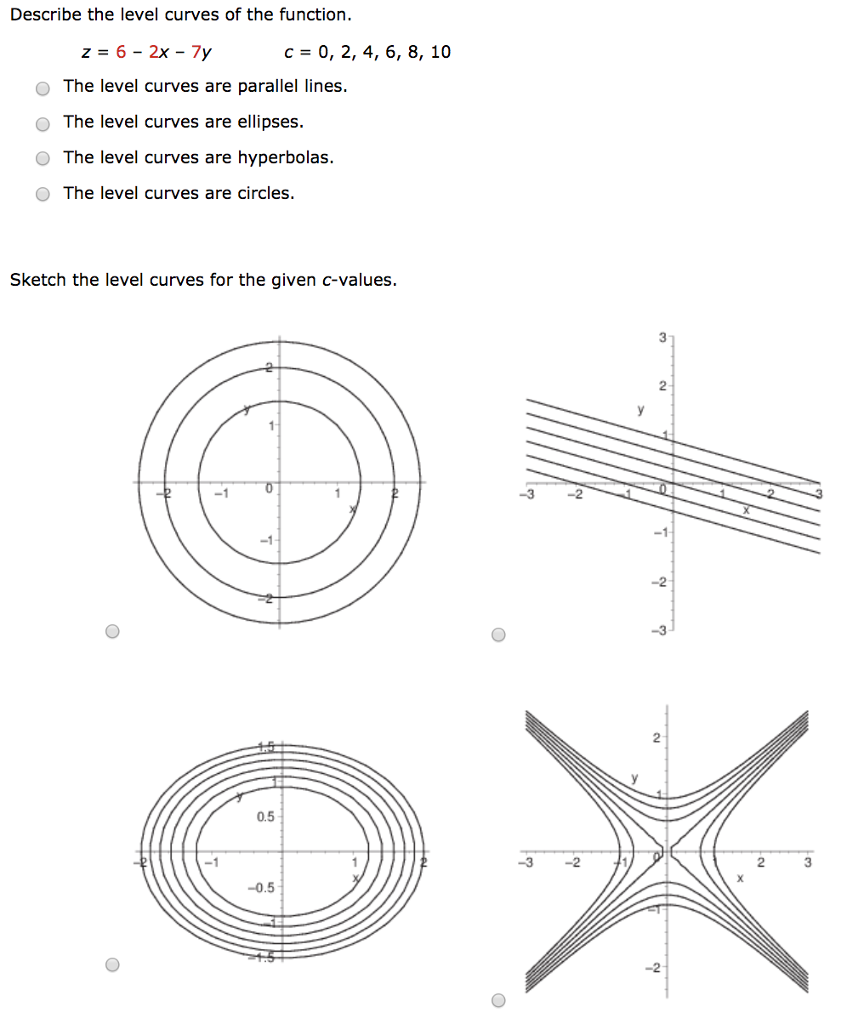
Solved Describe The Level Curves Of The Function Z 6 2x 7y Chegg Com

The function f = xy 15 Draw the contour map for f = xy with level curves f = has convex level curves (hyperbolas) 2, 1,0, 1, 2 Describe the surface 31 The hours in(a) Two different level curves of a function f(y) can intersect (b) For every function f(x,y) and every real number K, the level curve f(x, y) = K exists (and is nonempty) (c) If f is a function of x and y, and fwy = 0, then either fo = 0 or f = 0

Sketch level curves of a function

Sketch level curves of a function-Definition The level curves of a function f of two variables are the curves with equations f (x,y) = k, where k is a constant (in the range of f ) A level curve f (x,y) = k is the set of all points in the domain of f at which f takes on a given value k In other words, it shows where the graph of f has height kUh, which content the pond?

F 2 Figure 10 Shows A Constraint 9 X Y 0 And The Level Curves Homeworklib

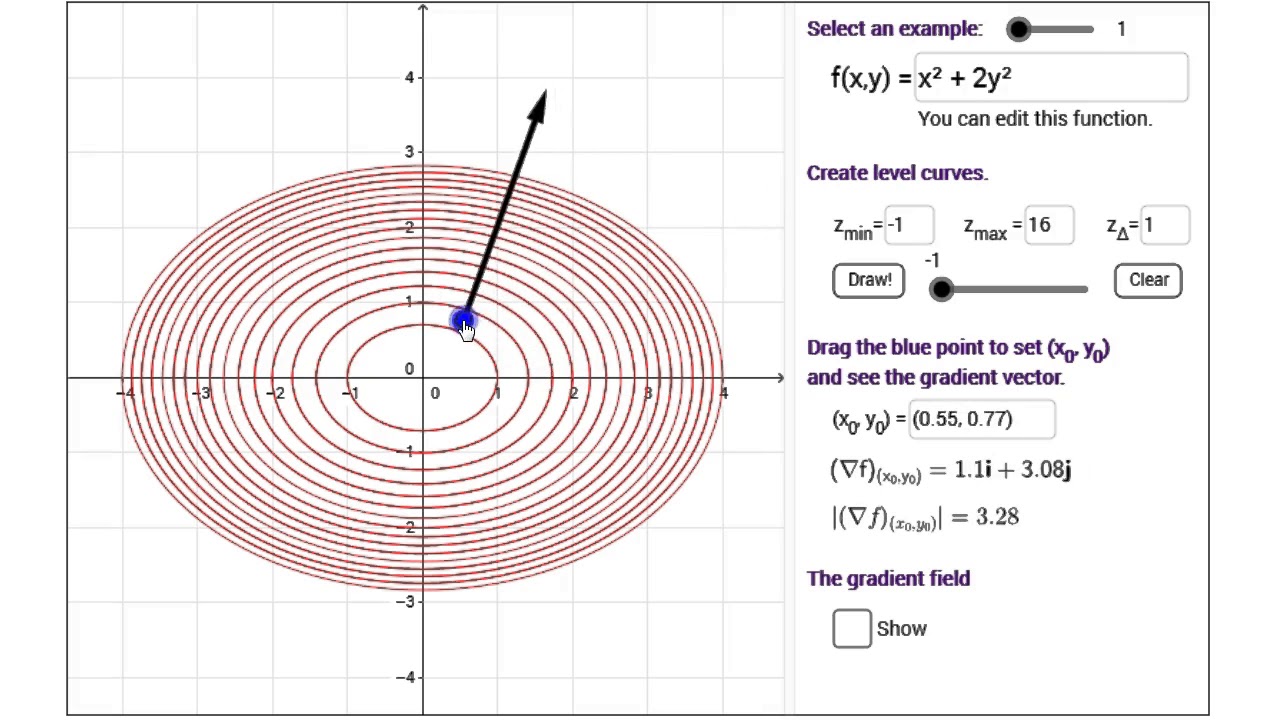
Level Curves So far we have two methods for visualizing functions arrow diagrams and graphs A third method, borrowed from mapmakers, is a contour map on which points of constant elevation are joined to form contour curves, or level curves A level curve f (x, y) = k is the set of all points in the domain of f at which f takes on a given value kReturning to the function g (x, y) = 9 − x 2 − y 2, g (x, y) = 9 − x 2 − y 2, we can determine the level curves of this function The range of g g is the closed interval 0, 3 0, 3 First, we choose any number in this closed interval—say, c = 2 c = 2 The level curve corresponding to c = 2 c = 2 is described by the equationSketch some level curves of the function Solution First, let z be equal to k, to get f(x,y) = k Secondly, we get the level curves, or Notice that for k>0 describes a family of ellipses with semiaxes and Finally, by variating the values of k, we get graph bellow (Figure 3), called, level curves or contour map Firgure 3 Level curves of f(x,y)

A level set L r ( d ) {\displaystyle L_ {r} (d)} of this function consists of those points that lie at a distance of r {\displaystyle r} from the origin, that make a circle For example, ( 3 , 4 ) ∈ L 5 ( d ) {\displaystyle (3,4)\in L_ {5} (d)} , because d ( 3 , 4 ) = 5 {\displaystyle d (3,4)=5}The authors construct a meromorphic function of bounded characteristic whose level set for 1 contains a sequence of closed Jordan curves which surround the origin and which converge toTHE LEVEL CURVES OF HARMONIC FUNCTIONS(1) BY LEOPOLD FLATTO(2), DONALD J NEWMAN(2) AND HAROLD S SHAPIRO(3) I Introduction In this paper we study the level curves of harmonic functions, ie, curves F for which there exists a harmonic function u(x,y) vanishing on F but not identically A necessary condition is that F be an analytic curve and,